 The website is in construction. New files will be uploaded as soon as they are available.
The website is in construction. New files will be uploaded as soon as they are available.
Felix Klein
Düsseldorf 1849 - Göttingen 1925

- Youth and University Studies
- The Early Academic Career
- Scientist and "Fachpolitiker"
- The Reform Agenda
- The Work for ICMI
- Essential bibliography
- Publications on the Teaching of Mathematics
- Obituaries
- Publications on Klein
Youth and University Studies
Felix Christian Klein was born in Düsseldorf, on April 25th, 1849. Düsseldorf, seat of the provincial government of the Prussian Rhineland and a centre of industrialization in Prussia, was - contrary to other parts of the Rhineland - of dominantly Protestant religion. His family was Protestant, too. Caspar Klein, Felix's father, acted as secretary to the provincial governor. His mother, Sophie Elise Klein, descended from a draper's family in Aachen. After elementary instruction at home by his mother, the six-year old boy entered a private school. He left it already two and a half years later and entered in 1857 the Gymnasium in Düsseldorf. Traditionally, one uses to ascribe to German Gymnasia a one-sided cultivation of classical education. This is not correct, however, for the Gymnasia in Prussia since their consistent reform in the 1810s and 1820s and in particular not for the Rhineland - at least until the 1850s. In fact, mathematics teaching was well represented at the Gymnasium in Düsseldorf and always taught by several qualified teachers. His solutions of the mathematics problems in the Abitur exam showed him as well instructed (Fischer 1985). And the Abitur-Zeugnis attributed to him equal qualities in the humanities and in mathematics and the sciences (Lorey 1926, 149).The original curricular conception of the Prussian educational reforms had been that of a complementary unity of three basic components of human knowledge: languages, history and geography, and mathematics and the sciences. Klein seems to have been profoundly moulded by this organic view; he always strove to overcome one-sided or dichotomic conceptions and to unravel essential correspondences and relations. His studies at the University of Bonn, which he began at the unusually early age of 16 and a half years, in 1865, document this desire of an all-embracing knowledge: he studied not only mathematics, but also all the natural sciences. This was possible thanks to the extraordinarily well organized studies at Bonn for the sciences: the Seminar für die gesamten Naturwissenschaften with its five sections for physics, chemistry, geology, botany and zoology offered a coherent and systematic program of theoretical and practical studies.

Klein as a young professor in Leipzig
As a result of Plücker's premature death in May 1868, Klein, who had closely assisted him in elaborating his new work on Liniengeometrie, was charged by Alfred Clebsch to elaborate the missing parts of its second volume. This new cooperation with Clebsch helped him produce the conception for a doctoral thesis, on a general form of Linienkomplexe of the second degree.
The Early Academic Career
In December 1868, less than twenty years old, he obtained the doctoral degree, examined by Lipschitz - and without a previous exam for a Gymnasium teacher licence, as was still the custom.
To perfect his mathematical qualifications, Klein moved to Göttingen and studied there from January to August 1869 with Clebsch who was about to establish a mathematical school rivalling that of Berlin. Klein was keen, however, to study in Berlin as well, and spent there the next months, until April 1870. He attended the highly specialized Mathematisches Seminar and a lecture by Kronecker, but did not want to attend a lecture by Weierstrass, not liking his dominant position. In Berlin, Klein got to know Sophus Lie and their cooperation became highly important for him. In April 1870, the two young men set up to Paris to study there new developments in French mathematics. It was the first time since the 1820s and since Dirichlet and Plücker that a German mathematician thought it worthwhile to study in France. The outbreak of the German-French war of 1870/71 in July ended, however, this research stay. Klein, patriotically minded, volunteered for the army, but served in a medical corps. Infected by typhus, he had to leave the army and used the time of recovery to prepare his Habilitation. He obtained this degree in January 1871 and acted in the following time in Göttingen in the characteristically German position of a Privatdozent.
He did not have to wait long in this intermediary position. Distinguished by already impressing geometric publications he obtained the position of full professor in October 1872, when only 23 years old, at the university of Erlangen. Mathematics had become modernized in Bavaria only from the late 1840s on, and there were but a few students in mathematics by that time. Klein would, however, learn the differing status of mathematics in the various institutional situations which it experienced in the numerous German states. His first position is for always tied with one of his major achievements, the famous Erlanger Programm - innovating and generalising the study of geometrical transformations.
It was unnoticed for a long time, however, that Klein set out at the same time a second "Erlanger Programm": It was traditional practice not only to deliver a scientific paper upon assuming a chair as full professor, but also to deliver an inaugural address, the Antrittsrede. In this speech, Klein demonstrated his profound interest in educational reform: he propagated a vision of uniting classical and realist secondary schools, which were organised in a particularly dichotomous manner in Bavaria (Rowe 1985). Clearly, this vision corresponded to the original ideal of Prussian neohumanist education.
Moreover, Klein developed in Erlangen skillful managerial qualities which should become characteristic of his professional activities: due to the untimely death of his second supervisor, Clebsch, in November 1872, he not only entered the editorial team of Mathematische Annalen, the journal established by Clebsch rivalling the Crelle journal, but had also to take over the leadership of the Clebsch school, so that several of the members rallied to stay with him in Erlangen (e.g., A. Voss, F. Lindemann, A. Harnack).
In 1875, Klein accepted the challenge to move to the technical college of Munich, with a considerable number of students studying mathematics, albeit as a service subject. He devoted himself intensely to assure a sound formation in the fundaments of mathematics for these students; in addition, he also looked after the few students specializing in mathematics, since there was the special situation in Bavaria where future teachers for the technical and realist schools were trained at the technical college. And Klein enjoyed coming into contact with technical sciences and their practitioners, in particular Carl von Linde.
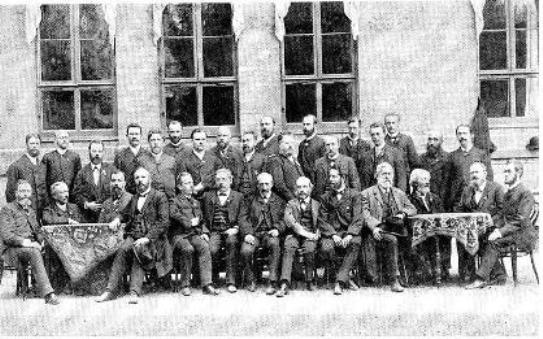
The founders of the Deutsche Mathematiker-Vereinigung in Bremen in 1890
Scientist and "Fachpolitiker"
In 1880, Klein accepted still another appointment, as professor of geometry at Leipzig University, in Saxonia. Mathematics at Leipzig had always maintained an independent and serious position within German universities. It was in Leipzig that Klein devoted himself to even more systematic efforts for improving the quality of studies for all mathematics students and succeeded in establishing decisive innovations: an independent building for mathematics with lecture halls, rooms for Übungen, for the collection of models, and for the newly established Mathematisches Seminar and - most notably - for a specialised library.
It was in Leipzig, too, that Klein - hitherto known mainly for his works on geometrical problems, in particular the icosaedron - worked intensely on analytic problems, prompted by elliptic functions involved in treating the icosaedron. Developing what he called "automorphe Funktionen", he entered into a fierce competition with Poincaré who worked on them, too, calling them "fonctions fuchsiennes". Although he was able to elaborate a key theorem for this theory, Klein suffered in 1882 a nervous breakdown and needed so long to recover that Poincaré surpassed him, making it impossible to catch up with him again and so achieving definite leadership. Klein continued up to the 1890s in productive research on the theory of functions, but he engaged more and more in activities as "Fachpolitiker".
The platform for such activities was provided to him by his call in 1886 to Göttingen - hence, as a successor to Riemann and to his supervisor Clebsch -, since Göttingen now belonged to Prussia, and Prussia as the dominant state within the confederation Deutsches Reich offered ample opportunities for policy making, including those outside the realm of education. It were calls to new, research oriented US universities and the alliance which he forged with Friedrich Althoff - the almost omnipotent Ministerialdirektor in the Prussian ministry -, which resulted in his obtaining the dominant position in the German mathematical community. The foundation of the Deutsche Mathematiker Vereinigung in 1890 still met resistance from the opposing Berlin school, but in the long run they were not able to resist integration into its dynamic (Hashagen 2003).
In 1896, Klein succeeded in having David Hilbert appointed to the other mathematical chair in Göttingen. Together, Hilbert and Klein achieved the transformation of their setting into the world centre of mathematics. Students and scientists from all the countries made the pilgrimage to this mathematical Mekka. Numerous new positions for mathematicians were established at this quasi-department, and in 1904 the first chair for applied mathematics was created, with Carl Runge as its first professor.
The Reform Agenda
One key issue in his agenda was the strengthening of relations of mathematics to its applications in the sciences and in technology. A first issue in this was the promotion of applied mathematics within the mathematical community, but also institutionally. This implied a redefinition of the mathematics to be taught at the technical colleges, which in the meantime aspired to become equivalent in status to the universities - a problematic he knew well since his own teaching at the Munich Polytechnic.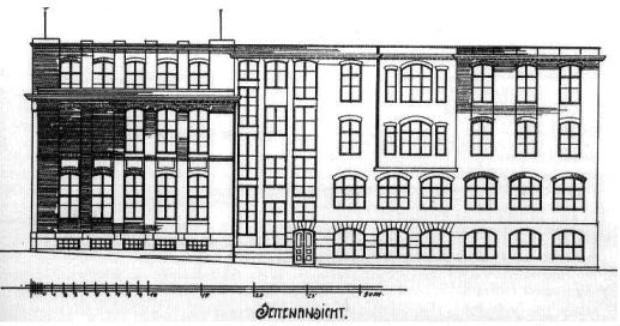
The plan for the Mathematical Institute in Göttingen, as projected by Klein in 1909
A next step taken for this issue was unprecedented: Klein forged an alliance with leading German industrialists to promote his program. They established in 1898 the Göttinger Vereinigung für angewandte Physik, broadened in 1901 to "und Mathematik". The association not only sponsored and co-financed research activities in Göttingen but also helped establish new buildings and institutes, most notably the new Physics Institute in 1904. Klein's dream was to use the means of the association to erect an analogous building and institute for mathematics. Resistance from the ministry of finance prevented its realization at first, then followed by WW I and, eventually, inflation. It was only after Klein's death and thanks to the Rockefeller Foundation that the first impressive building for a Mathematical Institute was inaugurated in 1929, in Göttingen.
The major issue was, however, to modernise mathematics teaching. Klein deplored what he used to call the "double gap": the discontinuity between school mathematics and university mathematics and the double forgetting of the respective knowledge: first one had to forget school mathematics upon beginning one's university studies and later as a teacher one had to forget university mathematics and return to school mathematics. In the 1890s, Klein developed many initiatives to improve teacher education, such as establishing summer schools as inservice-training and by cooperating with the teachers association, the Deutscher Verein zur Förderung des mathematischen und naturwissenschaftlichen Unterrichts. His lectures Elementary Mathematics from an advanced viewpoint, published in three volumes, became a universally adopted textbook for methodological formation of mathematics teachers, still serving as a model today.
Klein followed through his insight that a reform of university mathematics necessitated taking an even more extensive system into consideration: the school system as the basic foundation for higher education. He began, therefore, to interest himself in the improvement of teacher education. By so doing, he hoped to reverse the trend toward one-sidedly formal, abstract approaches to mathematics instruction by promoting practical instruction and the development of spatial intuition. An early breakthrough in this pursuit, although still realized by the traditional method of administrative decrees from "on high," took place in 1898. In that year new regulations for the examination of prospective teachers in Prussia were introduced. These created a second teaching license for applied mathematics, thus complementing the traditional one, which was thereafter referred to as the examination in "pure" mathematics. The new regulations also enabled the Prussian technical colleges to introduce disciplinary studies in mathematics for the first time. Henceforth, future mathematics teachers were allowed to spend three terms studying at these colleges. At the same time, the enlarged university curriculum made it possible to establish positions for applied mathematics at some of these institutions, thereby breaking up the monopoly that pure mathematics once enjoyed there.
The new university-like status attained by the technical colleges in 1899 as well as the imminent recognition of all three types of nine-year secondary schools as entitled to have their graduates admitted to institutions of higher education posed new structural problems, however, for mathematics within these institutions. Should the graduates of the modernist schools (Realgymnasien and Oberrealschulen) be directed only towards the Technische Hochschulen or to the universities as well? If the former course were to be adopted, this would imply acceptance of the Bavarian model with its bifurcated educational system and consequent split approach to mathematics instruction.
Instead of assimilating and integrating the technical schools (Realschulen) into the Gymnasien and the technical colleges into the universities, as he had proposed earlier, Klein now favoured the full and independent development of the Realschulen (which had undergone a remarkable process of transformation throughout the 19th century since their beginnings as vocational schools of low status), and the Technische Hochschulen (whose transformation had been almost as profound). Another consequence was that the mathematical curricula in all the various secondary and tertiary institutions would have to be redefined in order to make the free transition from each school type to both higher education types. Thus by 1900, Klein's views had evolved to the point that he now recognized that an effective and stable reform of the subsystem of higher education required changes that went beyond this institutional plane. A new approach to teacher education was needed; but beyond this the link to the underlying school system had to be reformed, in particular, by reforming the mathematical curricula in the secondary schools.
Klein proposed a radical reform. Since the mathematical courses at the technical colleges consisted of a basic preparatory "general" part and an advanced or higher part, he recommended that the basic studies be transferred to the preparatory schools, i.e. the secondary schools, and that only the advanced studies should remain as part of the college curriculum. However, they would be reformed and taught not as independent branches of knowledge, but rather "in permanent touch with the requirements of the students of mechanical and construction engineering.... Mathematics at the colleges can thus become once again what it ought to be: a specific power pervading the whole." Transferring the preparatory courses to secondary school would help create a firm mathematical basis for the pursuit of college studies, due, in particular, to the "benevolent coercion of school," as opposed to a university environment where academic freedom reigned. But which subjects were to be considered as part of the basic core of this new secondary mathematical curriculum? According to Klein, this core would include analytical geometry, but primarily differential and integral calculus!
In private letters to close friends and co-workers, Klein in fact always stressed that the key to his reform plans was to solve the question of mathematical preparation for the technical colleges. It is evident that the most complicated part of his proposal concerned the changes that would be required in school mathematics, particularly since the secondary schools had dropped analytical geometry and calculus from their curriculum more than a half-century earlier.
The Ministry refused to decree the desired curricular changes from above. Instead, they advised Klein to organise the introduction of the curricular changes "from below" by enlisting the support of appropriately trained teachers who could act as agents for the implementation of the reforms in selected schools. From 1902 on, Klein began an intense and thorough study of the state of mathematics education in order to discover the pivotal issues that might activate mathematics teachers. Having familiarized himself with some of the main problems facing mathematics teaching, in the schools, he proceeded to coin the key phrase that would hereinafter serve as the slogan for his reform program, but which also helped to convey the impression this program was motivated exclusively by a desire to improve education within the schools. This was the famous notion functional reasoning, or - to say it more concretely - the idea that the function concept should pervade all parts of the mathematics curriculum. Walther Lietzmann (1880-1959), Klein's principal assistant in organizing the reform movement later noted that the success of the reform movement depended on finding a fundamental idea that would serve as a rallying point and which at the same time would automatically carry the calculus into the Gymnasium curriculum. That pivotal rallying point was the concept of function, which according to Klein's program would already be introduced in the lower grades (Lietzmann 1930, 255). This slogan of functional thinking in hand, Klein began gathering support for this reform movement from below. He succeeded in triggering an enormously broad and dynamic reform movement among mathematics teachers of all school types and German states. Its most visible and seminal document is known as the "Meraner Reform" or the Meran syllabus, named after the syllabus proposed by a committee for all the three school types, presented at a meeting of the DMV in the then Austrian town Meran in 1905.
The Work for ICMI
When in 1908 the Fourth

Liebermann's painting of Klein 1912
Klein invested restlessly an enormous activity to meet this deadline. Being forced to stay 1911/12, for one year, in a sanatorium, due to serious health problems, in Hahnenklee in the Harz mountains, he managed to have meetings with his German assistants and with foreign colleagues. During the stay in Hahnenklee, Max Liebermann executed his famous painting of Klein, now located in the Göttingen mathematical institute.
The culmination of IMUK work was reached at the 1914 IMUK session in Paris where the two topics presented corresponded to the two main issues in Klein's reform agenda: the mathematical training of the engineers and "The Evaluation of the Introduction of Differential and Integral Calculus into the Secondary Schools". This was the topic that Klein prepared more carefully than any other. He not only helped design the international questionnaire that dealt with this matter, but he also chose its coordinator and reporter: Emanuel Beke, a Hungarian scholar.

The Paris meeting took place shortly before the outbreak of WW I, which should change everything. Neither the projected Congress of 1916, nor the last international thematic study, on the training of mathematics teachers, were realized. In October 1914, Klein signed, together with 92 other German scholars and intellectuals, the disastrous "Aufruf an die Kulturwelt" which denied any war crimes committed by the German army in Belgium, claimed a German cultural mission in Europe and backed German militarism.

Preparatory notes by Klein for a meeting with Lietzmann.
Issue no. "3" was the forthcoming IMUK meeting
at Heidelberg which had to prepare the Congress at Paris
Issue no. "3" was the forthcoming IMUK meeting
at Heidelberg which had to prepare the Congress at Paris
Klein retired in 1913, after his long illness, but not only did he remaine active in the IMUK work; he even engaged in new fields: having earlier on promoted research on history of mathematics and related publications, he gave now lectures on the history of mathematics in the nineteenth century. Later, these lectures became published: the seminal Vorlesungen über die Entwicklung der Mathematik im 19. Jahrhundert (1926/27). Together with Hilbert, he had worked on the mathematical aspects of the theory of special and general relativity.
On June 22, 1925, Klein died, in Göttingen.
Essential bibliography
F. KLEIN, Gesammelte mathematische Abhandlungen. Hrsg. von Robert Fricke et al. (Berlin: Springer, 1921-1923)
Vol. 1, Liniengeometrie. Grundlegung der Geometrie zum Erlanger Programm. Hrsg. von R. Fricke u. A. Ostrowski. 1921.
Vol. 2, Anschauliche Geometrie. Substitutionsgruppen u. Gleichungstheorie zur mathematischen Physik. Hrsg. von R. Fricke u. H. Vermeil. 1922.
Vol. 3, Elliptische Funktionen, insbes. Modulfunktionen. Hyperelliptische und Abelsche Funktionen. Riemannsche Funktionentheorie und automorphe Funktionen. Anh. Hrsg. von R. Fricke, H. Vermeil u. E. Bessel-Hagen. 1923.
F. KLEIN, Das Erlanger Programm: Vergleichende Betrachtungen über neuere geometrische Forschungen. (Leipzig: Akad. Verlagsges., 1974). [French and Italian transl.]
F. KLEIN, Funktionentheorie in geometrischer Behandlungsweise. Vorlesung, gehalten in Leipzig 1880/81; mit 2 Original-Arbeiten von F. Klein aus dem Jahre 1882. Hrsg., bearb. u. komm. von F. König (Leipzig: Teubner, 1987).
F. KLEIN, Vorlesungen über das Ikosaeder und die Auflösung der Gleichungen vom fünften Grade [Reprogr. Nachdr. der Ausg. Leipzig, Teubner, 1884]. Hrsg. mit einer Einf. und mit Kommentaren von Peter Slodowy (Basel: Birkhäuser, 1993). [Engl. transl.]
F. KLEIN, Einleitung in die analytische Mechanik: Vorlesung, gehalten in Goettingen 1886/87. Hrsg. und bearb. von E. Dietzel u. M. Geisler. Mit einem Beitrag von F. König. (Stuttgart: Teubner, 1991).
F. KLEIN, Riemannsche Flächen. Vorlesungen, gehalten in Göttingen 1891/92. Hrsg. und kommentiert von G. Eisenreich u. W. Purkert. (Leipzig: Teubner Springer [in Komm.]), 1986).
F. KLEIN, Einleitung in die höhere Geometrie. Vorlesung, [Bearb.] Friedrich Schilling, Göttingen. Faksimile-Druck:
Vol. 1, Wintersem. 1892/93. 1893
Vol. 2, Sommersem. 1893 . 1893
F. KLEIN, Vorlesungen über die hypergeometrische Funktion: [gehalten an d. Univ. Göttingen im Wintersem. 1893/94]. Ausgearbeitet von Ernst Ritter. Hrsg. u. mit Anm. vers. von Otto Haupt]. - Reprint [d. Ausg. Berlin 1933] (Berlin: Springer, 1981).
F. KLEIN, Vorlesungen über die Theorie der elliptischen Modulfunktionen. [Bearb.] Robert Fricke (New York: Johnson Teubner). Repr. of Leipzig 1890 - 1892:
Vol. 1, Grundlegung der Theorie. 1966.
Vol. 2, Fortbildung und Anwendung der Theorie. 1966.
F. KLEIN, Lectures on mathematics: delivered from Aug. 28 to Sept. 9, 1893 before members of the congress of mathematics held in connection with the world's fair in Chicago at Northwestern Univ., Evanston, Ill. Reported by Alexander Ziwet (New York: Macmillan, 1894).
F. KLEIN, Über lineare Differentialgleichungen der zweiten Ordnung. Vorlesung, gehalten im Sommersem. 1894. Ausgearb. von F. Ritter (Göttingen, 1894).
F. KLEIN, "Über Arithmetisierung der Mathematik", Nachrichten der Königlichen Gesellschaft der Wissenschaften zu Göttingen, Geschäftliche Mitteilungen, 1895, Heft 2.
F. KLEIN, Vorlesungen über die Theorie der automorphen Funktionen. Bearb. Robert Fricke (New York: Johnson Teubner): Repr. of Leipzig 1897 - 1912
Vol. 1, Die gruppentheoretischen Grundlagen. 1965.
Vol. 2, Die funktionentheoretischen Ausführungen und Anwendungen. 1965.
F. KLEIN; A. SOMMERFELD, Über die Theorie des Kreisels. Hefte 1-4 (Leipzig: Teubner) [Engl. transl.]:
Vol. 1, Die kinematischen u. kinetischen Grundlagen der Theorie . - 3. Aufl. 1923
Vol. 2, Durchführung der Theorie im Falle des schweren symmetrischen Kreisels. 2. Aufl. 1921
Vol. 3, Die störenden Einflüsse. Astronomische und geophysikalische Anwendungen. 2. Aufl. 1923
Vol. 4, Die technischen Anwendungen der Kreiseltheorie. 1910.
F. KLEIN, Vorlesungen über nicht-euklidische Geometrie. Für den Druck neu bearb. von W. Rosemann. - Reprint Berlin 1928: (Berlin: Springer, 1968).
F. KLEIN, Vorlesungen über die Entwicklung der Mathematik im 19. Jahrhundert (Berlin: Springer):
Vol. 1 (1926) [English translation: 1979]
Vol. 2 Die Grundbegriffe der Invariantentheorie und ihr Eindringen in die mathematische Physik . 1927.
Materialien für eine wissenschaftliche Biographie von Gauss. Gesammelt von F. Klein et al. 5 vol. (Berlin: Weidmann, 1911-1917).
Der Briefwechsel David Hilbert - Felix Klein: (1886 - 1918) . Mit Anm. hrsg. von Günther Frei (Göttingen: Vandenhoeck & Ruprecht, 1985).
Korrespondenz Felix Klein - Adolph Mayer: Auswahl aus den Jahren 1871 - 1907. Herausgegeben, eingeleitet und kommentiert von Renate Tobies et al. (Leipzig: Teubner, 1990).
Publications on the Teaching of Mathematics
F. KLEIN, Vorträge über ausgewählte Fragen der Elementargeometrie. Ausgearb. von F. Taegert. Eine Festschrift zu der Pfingsten 1895 in Göttingen stattfindenden 3. Versammlung d. Vereins zur Förderung des math. u. naturwiss. Unterrichts (Leipzig: Teubner, 1895).
F. KLEIN, "Über Aufgabe und Methode des mathematischen Unterrichts an den Universitäten", Jahresbericht der Deutschen Mathematiker Vereinigung, 7 (1899), 126-138.
F. KLEIN, Über angewandte Mathematik und Physik in ihrer Bedeutung für den Unterricht an den höheren Schulen: nebst Erläuterung der bezüglichen Göttinger Universitätseinrichtungen. Vorträge, gehalten in Göttingen, Ostern 1900, bei Gelegenheit des Feriencurses für Oberlehrer der Mathematik und Physik; mit einem Wiederabdruck verschiedener einschlägiger Aufsätze von F. Klein. (Leipzig: Teubner, 1900).
F. KLEIN, "Der Unterricht in der Mathematik", W. Lexis (ed.), Die Reform des Höheren Schulwesyens (Halle 1902),
F- KLEIN, Neue Beiträge zur Frage des mathematischen und physikalischen Unterrichts an den höheren Schulen: Vorträge gehalten bei Gelegenheit d. Ferienkurses für Oberlehrer d. Mathematik u. Physik, Göttingen, Ostern 1904. Gesammelt und hrsg. von F. Klein (Leipzig: Teubner, 1904).
F. KLEIN, Elementarmathematik vom höheren Standpunkte aus (Leipzig: Teubner/reprints: Springer, Berlin) [Engl. and Spanish transl.]
Vol. 1, Arithmetik; Algebra; Analysis. Reprint 4. Aufl. 1933. 1968.
Vol. 2 , Geometrie. Reprint 3. Aufl. 1925, 1968.
Vol. 3, Präzisions- und Approximationsmathematik. Reprint 3. Aufl. 1928, 1968.
F. KLEIN, Vorträge über den mathematischen Unterricht an den höheren Schulen. [Bearb.] Rudolf Schimmack (Leipzig: Teubner):
Vol. 1, Von der Organisation des mathematischen Unterrichts. 1907.
F. KLEIN, Universität und Schule: Vorträge auf der Versammlung Deutscher Philologen und Schulmänner am 25. September 1907 zu Basel (Leipzig: Teubner, 1907).
F. KLEIN, Aktuelle Probleme der Lehrerbildung: Vortrag auf der Versammlung d. Vereins zur Förderung d. mathemat. u. naturwiss. Unterrichts am 6. Juni 1911 zu Münster; Mit verschiedenen Anlagen. (Leipzig: Teubner, 1911).
Obituaries
RICHARD COURANT 1925, "Felix Klein", Jahresberichte der Deutschen Mathematiker-Vereinigung, 34, 197-213.
RICHARD COURANT 1925, "Felix Klein", Die Naturwissenschaften, 134, 765-772.
WILHELM LOREY 1926, "Felix Klein", Leopoldina, Berichte der Kaiserlichen Deutschen Akademie der Naturforscher in Halle, 1, 136-151.
M. WEBER 1925, "Felix Klein", Zeitschrift des Vereins Deutscher Ingenieure, 69, 1118-1119.
Publications on Klein
GERD FISCHER 1985, "Abitur 1865: Reifeprüfungsarbeit in Mathematik von Felix Klein", Der mathematische und naturwissenschaftliche Unterricht, 38, 459-465.
ULF HASHAGEN 2003, Walther von Dyck: (1856-1934); Mathematik, Technik und Wissenschaftsorganisation an der TH München , Stuttgart: Steiner.
K.-HEINZ MANEGOLD 1968, "Felix Klein als Wissenschaftsorganisator", Technikgeschichte, 35, 177-204.
KARL-HEINZ MANEGOLD 1970, Universität, Technische Hochschule und Industrie: ein Beitrag zur Emanzipation der Technik im 19. Jahrhundert unter besonderer Berücksichtigung der Bestrebungen Felix Kleins, Berlin: Duncker & Humblot.
HERBERT MEHRTENS 1990, Moderne - Sprache - Mathematik: eine Geschichte des Streits um die Grundlagen der Disziplin und des Subjekts formaler Systeme, Frankfurt am Main: Suhrkamp.
CONSTANCE REID 1978, "The Road not taken", Mathematical Intelligencer, 1, 1: 21-23.
DAVID E. ROWE 1985, "Felix Klein's, Erlanger Antrittsrede', a Transcription with English Translation and Commentary", Historia Mathematica, 12, 123-141.
DAVID E. ROWE 1985, "Essay Review: Felix Klein (Renate Tobies with Fritz König; Karl-Heinz Manegold; Lewis Pyenson)", Historia Mathematica, 12, 278-291.
DAVID E. ROWE 1986, "'Jewish mathematics' at Göttingen in the era of Felix Klein", Isis 77, 422-449.
DAVID E. ROWE 1989, "The early geometrical works of Sophus Lie and Felix Klein",The history of modern mathematic s, vol. I, Boston, MA, 209-273.
DAVID E. ROWE 1989, "Klein, Hilbert, and the Göttingen mathematical tradition", Osiris (2) 5, 186-213.
DAVID E. ROWE 1992, Felix Klein, David Hilbert, and the Göttingen mathematical tradition. New York, N.Y., City Univ. of New York, PhD thesis.
DAVID E. ROWE 1994, "The philosophical views of Klein and Hilbert",The intersection of history and mathematics, Basel, 187-202.
GERT SCHUBRING 1989, "Pure and Applied Mathematics in Divergent Institutional Settings in Germany: the Role and Impact of Felix Klein", The History of Modern Mathematics. Volume II: Institutions and Applications eds. David Rowe, John McCleary, Boston: Academic Press, 171 220.
GERT SCHUBRING 1999, "O primeiro movimento internacional de reforma curricular em matemática e o papel da Alemanha: um estudo de caso na transmissão de conceitos", Zetetiké, 7, no. 11, 29-50.
GERT SCHUBRING 2000, "Felix Kleins Gutachten zur Schulkonferenz 1900: Initiativen für den Systemzusammenhang von Schule und Hochschule, von Curriculum und Studium", Felix Klein und die Berliner Schulkonferenz des Jahres 1900, Der Mathematikunterricht, 46: 3, 62-76.
A. SHIELDS 1998, "Klein and Bieberbach: mathematics, race, and biology", The Mathematical Intelligencer, 10 (3), 7-11.
RENATE TOBIES 1981, Felix Klein , Leipzig: Teubner.
RENATE TOBIES 1992, "Felix Klein in Erlangen und München: Ein Beitrag zur Biographie", in S. S. Demidov et al. (eds), Amphora: Festschrift for Hans Wussing on the occasion of his 65th birthday, Basel- Boston- Berlin, 751-772.
JÜRGEN VON UNGERN-STERNBERG; W. VON UNGERN-STERNBERG 1996, Der Aufruf ''An die Kulturwelt!'': das Manifest der 93 und die Anfänge der Kriegspropaganda im Ersten Weltkrieg; mit einer Dokumentation, Stuttgart: Steiner.
Author
Gert Schubring
Institut für Didaktik der Mathematik,
Fakultät für Mathematik, Universität Bielefeld
gert.schubring@uni-bielefeld.de
